Particulate flows in industrial applications

Participants

AM 3

• Eberhard Bänsch
• Rodolphe Prignitz

External

• Wolfgang Peukert
• Jochen Schmidt

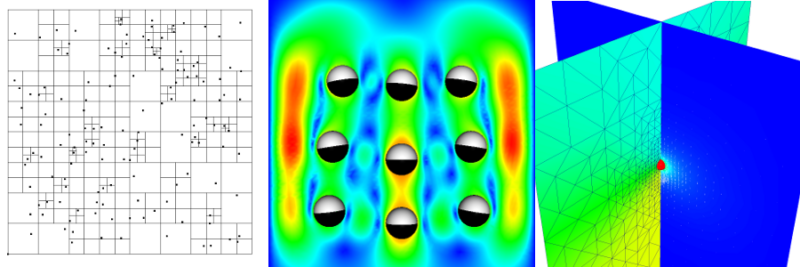
Description

The flow of particles in a fluid can be found in numerous industrial settings which use sedimentation, fluidisation and lubricated transport such as food processing, catalytic processing, slurries, coating, paper manufacturing, particle injection molding and filter operation. The ability to understand rheology effects of particulate flows is important for the design, operation and efficiency of the underlying processes. Despite the fact that particle technology is widely used, still it is an enormous experimental challenge to determine the correct parameters for the process used.

Our goal is to develop feasible algorithms and programs which are able to simulate particulate flows. For an industrial research project we need fast and accurate solutions. The result of our work will improve physical understanding of dispersions with a high volume fraction of particles.

For the simulation of such processes we consider a Newtonian fluid with rigid particles in our numerical model. The particles can interact through molecular or electrical forces. The method uses a finite-element discretisation in space and an operator-splitting technique for discretisation in time and has its basis in work by \citet{tesla}. However, rather than using a Lagrange multiplier, a subspace projection is used to couple the particle motion with the fluid motion. The advantage of this approach is the implicit treatment of the particle and the fluid velocity. Combined with local mesh refinement, the method results in a fast and accurate algorithm for the simulation of a huge number of particles in a flow field.