Interactive High-Performance Computing
Interactive high-performance computing (HPC)
Participants
AM 3
LS 10 (Informatik)
- Marco Heisig
Description
Relevant problems for high-performance computing are large-scale industrial problems, problems posed in high-dimensional spaces, high-precision calculations, large parameter studies and optimization problems or a combination of all those. Often these problems arise in domains which are not yet well-known, such that an exploratory approach becomes necessary for treating them effectively.
The standard HPC approach, which consists of using highly effective, but problem- and hardware-specific statically compiled software in a batch environment is ill-suited under these circumstances. Therefore we propose a new approach which embodies interactivity from the bottom up by using the interactive language Common Lisp which can compile to fast machine code on-the-fly.
On top of Common Lisp we have implemented the free software framework Femlisp for solving partial differential equation. A parallel object-oriented layer DDO/CL-MPI shields the Femlisp user/programmer from the underlying parallel machine.
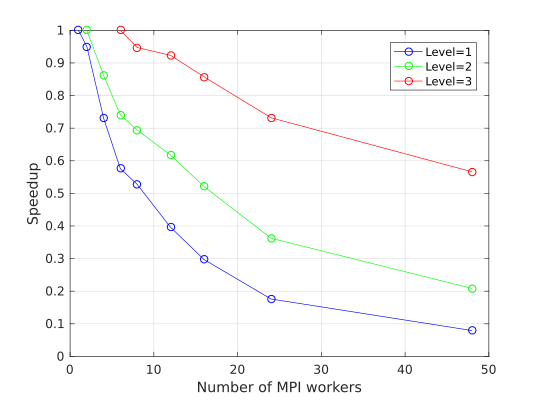
We have tested this setup for computing a homogenized coefficient for a 3D elasticity problem. The first image below shows some displacement vector field for a representative cell subjected to a certain stress situation. The second image shows a graph of the parallel speedup for this calculation on a Linux cluster.