Optimal control
Optimalsteuerung
|
The project focuses on control theory in order to study hybrid dynamical systems composed from the model hierarchy of gas networks up to the level of nonlinear iso-thermal Euler equations on pipelines and their interaction with discrete network components such as on/off-flaps, -valves and -compressors. Details Based on regularity and sensitivity results obtained in the first funding period the project now designs and investigates receding horizon methods that are able to take decisions based on optimality principles for the control of such systems also under uncertainties for example being applicable for the control of a gas network by valves in non stationary situations. |
|
Transregio 154: TP A03 (2014-2018) Mixed Discrete-Continuous Dynamical Systems with Partial Differential Equations The project focuses on control theory in order to study hybrid dynamical systems composed from the model hierarchy of gas networks up to the level of nonlinear iso-thermal Euler equations on pipelines and their interaction with discrete network components such as on/off-flaps, -valves and -compressors. Details The main line of research concerns mathematical foundations and shall answer questions for example regarding regularity and sensitivity of solutions for hyperbolic PDEs interacting with discrete events. The main challenges are the formulation of suitable solution concepts and their mathematical abstraction including the ability to topologize the instances and to find representations of sensitivities for events using adjoint-based methods. In case of state dependend switching, zeno effects are to be considered. Besides contributions to the above theoretical foundations, we expect by our study novel, locally convergent numerical methods by embedding the developed sensitivity fomulas into derivative based optimization algorithms in order to effentiently solve mixed-integer optimal control problems on the level of PDE gas-dynamics. |
|
Dr. Falk Hante (Erlangen), Prof. Günter Leugering (Erlangen) |
|
Feasibility: Robust Nodal controllability (2014-2017) We study optimal control problems with hyperbolic pdes and boundary data with stochastic influence. Nodal controls means that the control acts at a finite number of points in the network. |
| Prof. Dr. Martin Gugat (Erlangen), Prof. Dr. Rüdiger Schultz (Duisburg) |
|
EWave – Energy management water supply (2014-2016) The EWave project is concerned with the development of an decision support system for energy optimal management of water supply systems. At the moment, water supply is oriented towards security of supply. Based on increasing energy costs the BMBF-research program EWave has the goal to improve the energy efficiency of water supply. Therefore an energy management system should be developed. This system should be applied and tested at a water company for the first time. In the first step, it should serve as strategic planning tool for the plant management. It should be possible to examine basic considerations of the “plant operation mode” which should be used as short-term operating instructions. In a second step it is intended to use it as an operative decision support system. In the development of such an energy management system, various mathematical problems occur. First, a mathematical description of the supply network has to be modeled. On the one hand, the flow of water in the pipes is simulated. On the other hand, the systems of water treatment and distribution must be included in the model. An adequate modeling of these aspects leads to a high-resolution model of the water supply network. Partners in industry: Siemens AG, RWW und GreyLogix Aqua GmbH
|
|
Prof. Dr. Günter Leugering (Erlangen), Dipl.-Technomath. Maximilian Walther (Erlangen) |
|
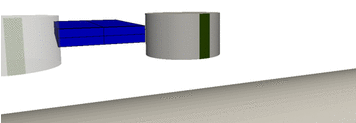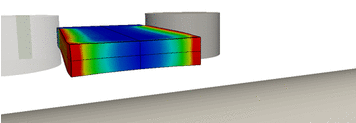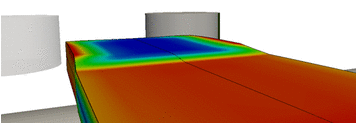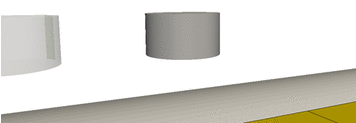
Model-Based Control of Slab Shapes in Hot Rolling Processes (2010-2013) We want to minimize unnecessary manufacturing costs arising in hot rolling processes by solving an optimal control problem that uses the trajectories of the rolls as control functions. We consider hot rolling processes, in which a metal solid body is deformed by several rolls in order to obtain a desired final shape. To minimize the cutting scrap and to ensure at the same time that this shape satisfies the required tolerances as accurately as possible, we formulate an optimal control problem where we use the trajectories of the rolls as control functions. The associated constraints defining the admissible set of the problem result from industrial specifications and technical limitations.
The motion and deformation of a solid body subject to different external forces can be described through the basic equations of nonlinear continuum mechanics, which are here coupled with an elasto-viscoplastic material model. This model is based on a multiplicative split of the deformation gradient into elastic and plastic parts and specifies the behavior of metals at high temperatures and high rates of deformation. Since we moreover assume that we can neglect the deformations of the rolls, we also have to add unilateral frictional contact boundary conditions and therefore end up with an evolutionary quasi-variational inclusion as weak form of the state problem. |
| Prof. Dr. Günter Leugering, Prof. Dr. Michael Stingl, Dr. Stefan Werner |
|
Control of System Dynamics in Gas and Water Networks (2009-2012) The dynamics in gas or water networks are governed by the isothermal Euler equations or the Saint Venant equations (shallow water equations), respectively, which form hyperbolic balance laws. For these networks we analyse (optimal) control and stabilization problems as well as the numerical application of the theoretical results. Efficient control of gas and water transportation networks plays an important role in industrial and municipal supply as well as in flood management. The gas flow through pipes can be controlled via compressors and valves, the water flow in canals can be controlled via pumping stations and flow gates. The picture shows a part of a compressor station (source: WINGAS). The dynamics in gas or water networks are governed by the isothermal Euler equations or the Saint Venant equations (shallow water equations), respectively, which form hyperbolic balance laws. For these networks we analyse (optimal) control and stabilization problems as well as the numerical application of the theoretical results. This project is a cooperative project between the RWTH Aachen University and the Friedrich-Alexander-University Erlangen-Nuremberg and is funded by the DFG Schwerpunktprogramm 1253. |
|
Prof. Dr. Günter Leugering (Erlangen), Prof. Dr. Martin Gugat (Erlangen), Dr. Markus Hirsch-Dick (Erlangen), Prof. Dr. Michael Herty (Aachen) |

 Transregio 154: TP A03 (2018-2022)
Transregio 154: TP A03 (2018-2022)